Physics of Fitness Fridays - Hula Hoops!
Hula hoops! They are a great combination of fun and fitness. But how do they work? And why can it be so difficult to keep one up?
Hula hoop motion is extremely complicated…what I’m going to talk about today is a very simplified view of what’s going on using force diagrams. (For you physics folks, I’m going to assume that your body is a point mass. I’m also going to assume that that point is not moving while it’s exerting its force on the hoop.)
Ready? Let’s get spinning!
There are three main forces acting on a hula hoop when you’re spinning it. The first force is gravity acting on the center of mass of the hoop. We denote this by the weight W, which is equal to the hoop’s mass m times the gravitational constant g.
Next, your body provides a normal force N outward against the side of the hoop each time your hip gives the hoop a little nudge. Because there is friction between your body and the hoop, a frictional force Ff pulls the hoop up. The force of friction is just the normal force times a constant u, called the coefficient of friction.
The normal force from your hips are what keep the hula hoop on a circular* path. Thus, we equate the normal force to the centripetal force Fc. The centripetal force is related to the angular speed w (you can think of it as “revolutions per second”) and radius of the hoop r by: Fc = m(w^2)r. Thus, N = m(w^2)r.
*(Note: Again, I’m assuming that your body is a point so that the hoop center moves on a circular path. In reality, the hoop moves around your waist in a “flower shaped” pattern—think of a spirograph.)
Since the hoop is not moving up or down your body, the force of friction is equal to the weight of the hoop: Ff = W, or mg = uN.
So let’s put the two equations together:
mg = uN = uFc = um(w^2)r
Which gives us an equation for the angular speed in terms of friction and hoop radius:
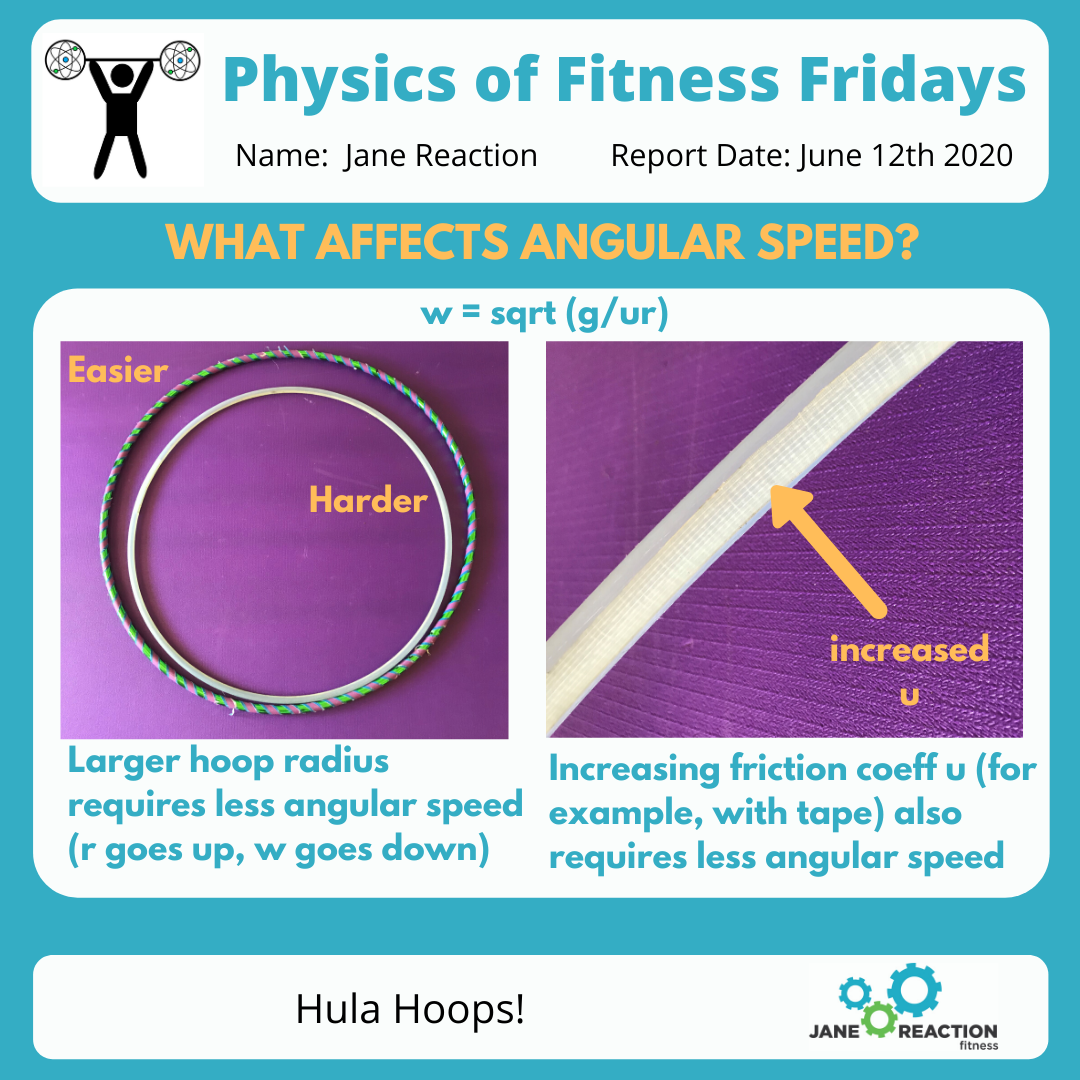
w = sqrt(g/ur)
When w is equal to this value, the forces balance and the hoop stays at your hip height. But what if you spin it faster or slower? According to the equations, if you spin the hoop faster, the centripetal force, normal force and force of friction will all increase. The force of friction will become greater than the weight of the hoop, and the hoop will move up your body.** If the hoop slows down, the hoop will start to fall.
As an adult, have you ever tried to hula hoop with a child’s hoop only to find that you’re miserable at hooping? Well, it wasn’t your fault- it was the hoop’s fault! If you look at the equation again for angular speed you’ll see why:
w = sqrt(g/ur)
As the radius r decreases, the angular speed you need to keep it up increases*. If you go online to buy a hoop, you’ll see “beginner’s hoops” that are in larger diameters. As your skill improves and you can move with more speed, smaller hoops are more accessible.
Another thing worth noting…as the coefficient of friction goes down, the speed needed to keep the hoop stable ALSO goes up. If you ever try to hoop in a fabric that’s slippery, you’ll quickly see how hard it can be to hoop. So wearing softer fabrics make it easier to hoop. (My old hooping instructor used to say, “hoop naked!” because your skin is “stickier” and helps keep the hoop up. I do not recommend trying that in public.) Another way to increase the coefficient of friction is to add a sticky tape on the inside of the hoop. This also increases u and makes hooping easier.
*(Note: Technically, it’s the difference between the radius of your waist and the waist of the hoop, not just the radius of the hoop itself—again, my explanation assumes you’re a point mass! Little kids can twirl their kid-sized hoops because their waists are smaller and the difference in radii is larger, not because they have super-human speed skills.)
So here are your takeaway hooping tips from a scientist:
1) If the hoop starts to fall, hoop faster to keep it up
2) If you’re having trouble hooping, try a larger hoop to make it easier
3) Don’t be too slippery when hooping! Increase friction between you and the hoop with your clothing (or no clothes?), or try adding tape!
As I mentioned, the above was a very simplified treatment of hoops. If you really want to make your head spin (see what I did there?), you can check out some of these technical treatments on the matter.
** Correction!
It was brought to my attention that my conclusion in panel five was a bit oversimplified. I concluded that if your speed is fast enough, that would make the hoop spin UP your body, overcoming gravity. More accurately, the hoop will stay at a constant height if your speed is higher than a minimum speed, or w >= sqrt(g/ur).
If the angular speed is equal or greater than this minimum value, the hoop is kept in place due to the force of static friction opposing gravity. If the angular speed goes below this value, then gravity will bring the hoop downward and the opposing force is due to kinetic friction. In the video below, you can see that the hoop stays at a set height until the speed slows down (about five seconds in) sufficiently enough that the hoop starts to drop.
So what can send the hoop upward? Mainly, the normal force provided by your hips is not completely horizontal; there is a slight vertical component. Depending on the speed of the hoop and the angle your body makes against the hoop, this can help drive the hoop upward. So the takeaway is that to keep the hoop up, you still want to hoop faster, but a little upward flip of the hip helps too!
Thanks to Rodney Cross from the University of Sydney for writing in and providing the video below!