Physics of Fitness Fridays - Weightlifting and Work

It’s Friday, so that means it’s time to discuss the physics behind another fitness topic. And this week, I’ve got some bad news. Despite all of your efforts at the gym, you’re just not working as much as you think you are.

Say whaaaaa?…

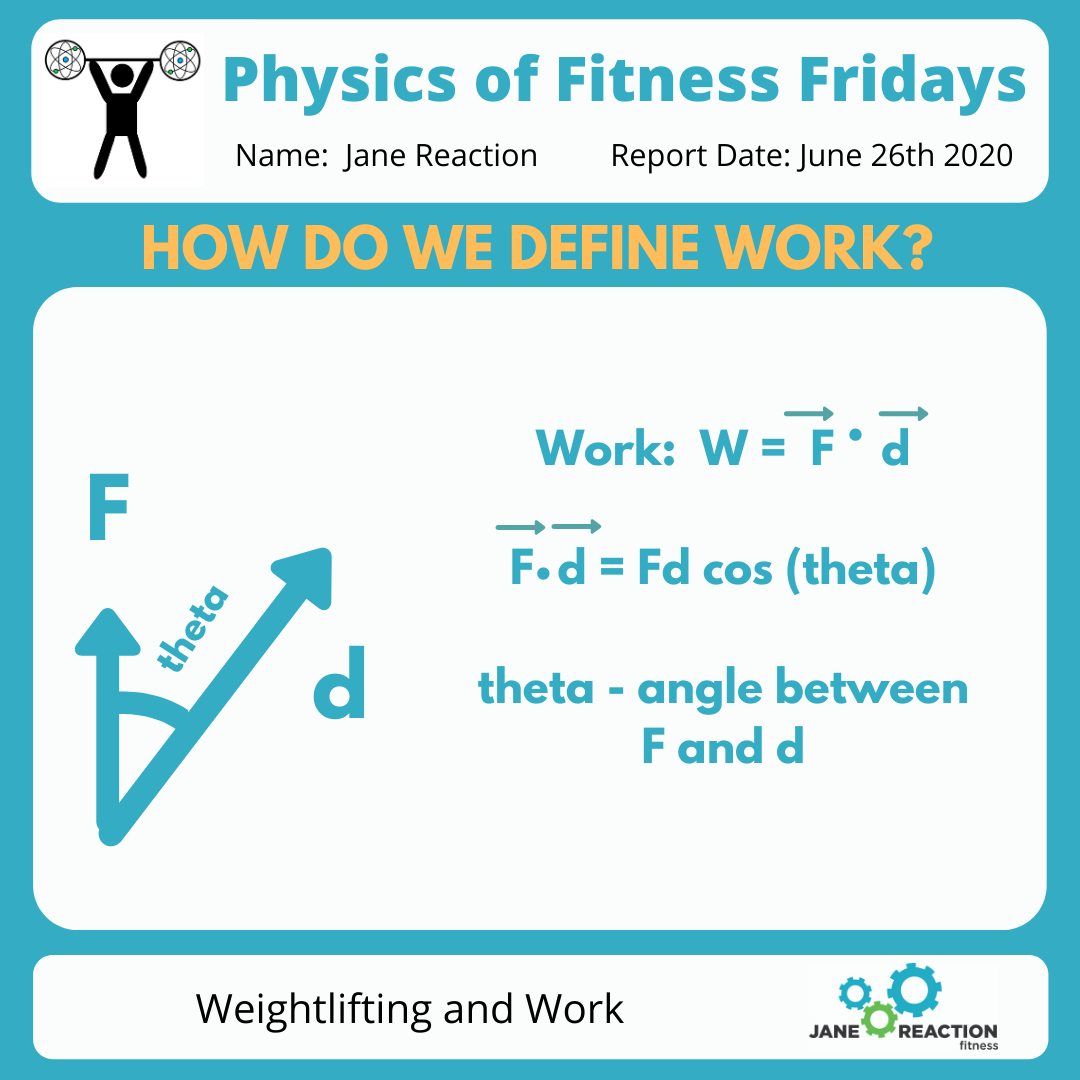
In physics, work is defined as the force applied over a given distance. This is represented by the dot product of force and distance (see equation on right). Force and distance are vectors, which means that they have a magnitude and a direction. So when we take the dot product of the two, we’re also looking at the angle between them.

So if we have a force F that is applied over a distance d, the angle theta between them affects the total magnitude of work done. This is defined as:

W = Fd cos(theta)

So if we have a force that is applied perpendicularly to the distance the object moves, there is no work performed because cos(90 degrees) = 0. If F and d are in the same direction, the work performed is just the product of the two values. And if the force and distance are in opposite directions, the work is negative.

You’re probably wondering what the hell I’m talking about. Let’s look at some examples…

When you are holding a weight, you are preventing that weight from falling to the ground. Thus you are applying an upward force to the weight that is equal and opposite to the force of gravity pulling the weight down, or simply F = mg, where m is the mass of the weight and g is the gravitational constant. If you pull that weight up (infinitely slowly, for reasons I won’t get into right now) and raise it a distance d, you perform an amount of work W = mgd. Note that your force and the distance moved are both pointing up.

Okay, so now let’s lower the weight back down. You’re still applying an upwards force. (If that confuses you, think about it- if you weren’t there, the weight would immediately drop to the ground.) But now, the weight is moving downward, and the force and distance are in opposite directions. Thus, by our work equation, you have done negative work on the weight, or -mgd.

Now let’s say you’re just holding the weight. It’s not moving, so in our work equation, d=0. That means….you are doing NO WORK on the weight!

What?!?

Now, let’s say you take your weight and carry it a distance d. Surely you’ve done work, right? I mean, the weight moved. But if you remember, here the force is pointing upward, but the distance moved is perpendicular to the force. So by physics standards, you have again done NO WORK.

Say it ain’t so!

So let’s summarize…when you lift a weight, you do positive work. When you lower a weight, you do negative work. That means that for one rep, you do a total of…no work?

When you’re holding a weight you’re doing no work, and when you’re carrying a weight you’re doing no work, and…and…WTF?!?

Exercising may seem as futile as me pushing against this wall, but don’t fret. As it turns out, in the physics world, work refers to mechanical work. This type of work is defined as the energy transfer between two objects. I’m pushing on this wall and nothing is happening, so from a physics standpoint I am doing no work. However, I can feel the tension and am clearly getting tired. That’s because I am converting glucose into energy and my muscle fibers are firing. I am doing physiological work.

Whew, that’s a relief! So you ARE doing work, just not from a physics standpoint. Which begs the question, “why do physicists define work in such a specific way?”

Because physicists are JERKS, that’s why.

Have a good week, and GET TO WORK!