Physics of Fitness Fridays: Rollin', Rollin', Rollin'

This week I want to discuss some fun things about the physics of rolling. Let’s roll out!

Rolling is the combination of pure translation and pure spinning.

For an outside observer, translation, rotation and rolling all look very different; with respect to the ground, each point on the wheel is moving at different speeds. Here I’ll look at three points: the top of the wheel, the center and the bottom. For translation, imagine the wheel skidding (across water or say, a sheet of ice). For pure translation, the wheel is sliding without any rotation. Thus, all three points of the wheel have the same linear velocity. For pure rotation (about the center), the top and bottom of the wheel will have equal and opposite linear velocities, while the center (being the axis of rotation) has no velocity; it’s just sitting there. For rolling, we can add the linear velocities of translation and rotation. Here the top of the wheel moves with a linear velocity of v from translation AND of rotation; it’s total velocity is 2v. For the center, it has a linear velocity v from translation. For the bottom of the wheel, the v from translation is canceled out by the v of rotation; that means that the bottom of the wheel is stationary with respect to the ground!

The linear velocity of the wheel, v, is linked to the angular velocity w by: v = wR, where R is the wheel’s radius.

v = wR means that, for two objects rolling with the same linear speed, the one will smaller wheels will have a larger angular speed. So if I ride a bike and skate at 10mph, the skate wheels are going to be spinning a heck of a lot faster (better have good bearings!)

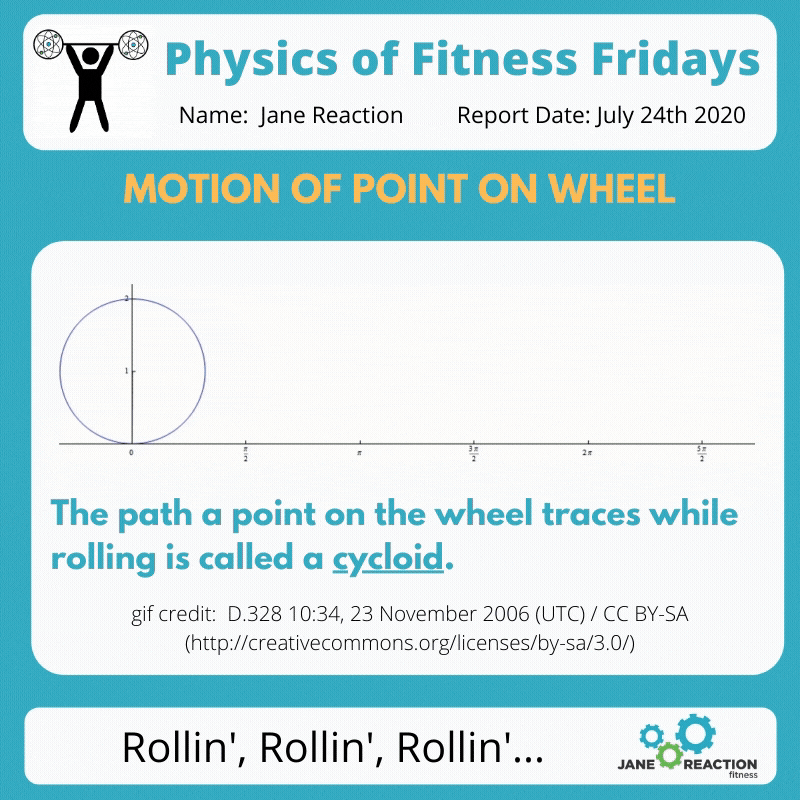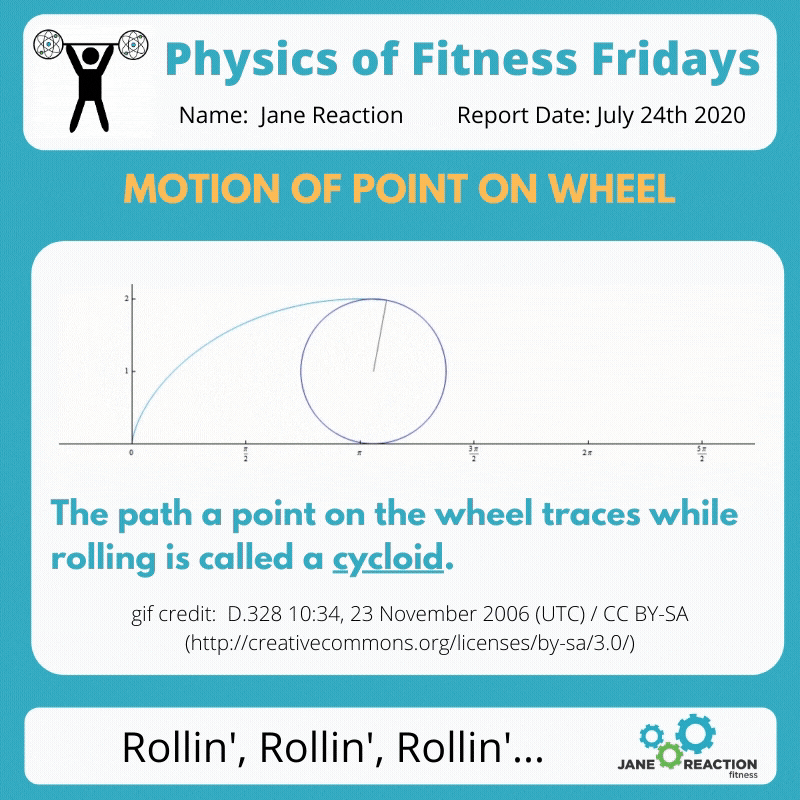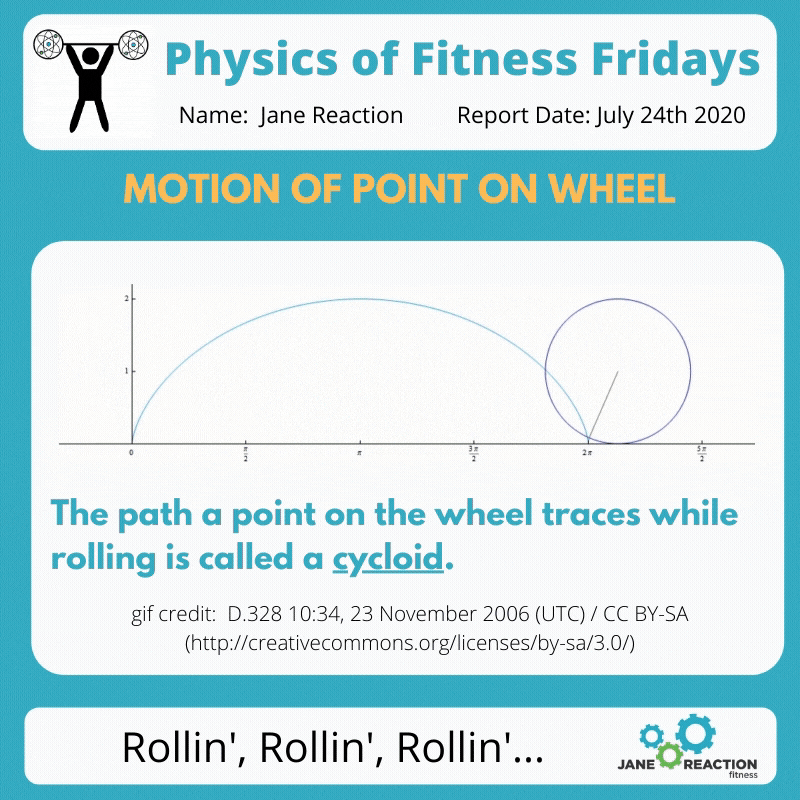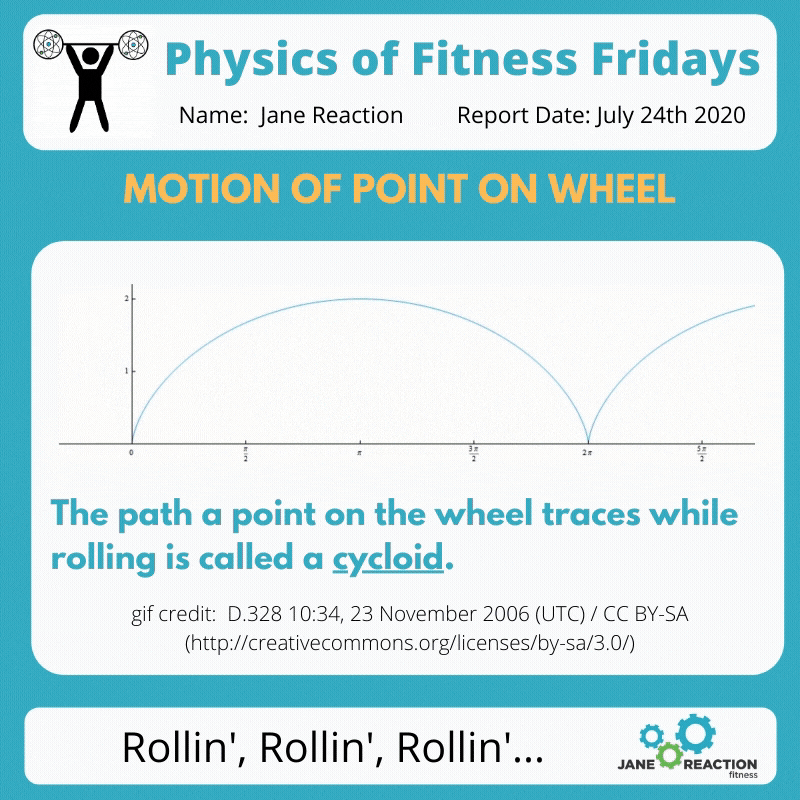
If you want to zone out, watch this gif that shows the path a point on a wheel makes as it rolls This path looks like a series of arcs and is called a cycloid.

Welp, that’s all I have for you this week. You may be wondering, “but Jane, is that it?” No, I have more, but if you want the juicy stuff you’ll just have to wait until next time. Sorry, that’s how I’m gonna roll…

See you later!